MTW European Type Trapezium Mill
Input size:30-50mm
Capacity: 3-50t/h
LM Vertical Roller Mill
Input size:38-65mm
Capacity: 13-70t/h
Raymond Mill
Input size:20-30mm
Capacity: 0.8-9.5t/h
Sand powder vertical mill
Input size:30-55mm
Capacity: 30-900t/h
LUM series superfine vertical roller grinding mill
Input size:10-20mm
Capacity: 5-18t/h
MW Micro Powder Mill
Input size:≤20mm
Capacity: 0.5-12t/h
LM Vertical Slag Mill
Input size:38-65mm
Capacity: 7-100t/h
LM Vertical Coal Mill
Input size:≤50mm
Capacity: 5-100t/h
TGM Trapezium Mill
Input size:25-40mm
Capacity: 3-36t/h
MB5X Pendulum Roller Grinding Mill
Input size:25-55mm
Capacity: 4-100t/h
Straight-Through Centrifugal Mill
Input size:30-40mm
Capacity: 15-45t/h
Spherical pendulum mill
Particle Size Reduction and Enlargement ScienceDirect
2019年1月1日 Quantification of the size reduction and enlargement process can be carried out using population balance equation (PBE) In this chapter, formulation and solution of PBE for All spherical pendulums are similar to each other Upon appropriate nondimensionalization of time and action, they are described by one and the same dimensionless LagrangianPlane and Spherical PendulumsA spherical pendulum is similar to a simple plane pendulum, except that the pendulum is not constrained to move in a plane; the mass \(m\) is free to move in two dimensions along the 412: The Spherical Pendulum Physics LibreTextsA spherical pendulum is a 2 degreeoffreedom mechanism consisting on a rod whose tip moves on the surface of a sphere It is common to use two angular coordinates to describe such a Full article: Modelling and control of a spherical pendulum via a
Spherical Pendulum, Actions, and Spin Universiteit Utrecht
The mathematical definition of a spherical pendulum assumes a mass point m, free to move on a sphere S 2 of radius r, in an external gravitational potential that depends linearly on aspherical pendulum of unit length swings from a xed point of support under the constant acceleration of gravity g (Figure 27) This motion is equivalent to a particle of unit mass M345 A16 Notes for Geometric Mechanics: Oct{Nov 2011In this chapter we treat the spherical pendulum as a constrained Hamiltonian system We derive Hamilton’s equations and show that there is an axial symmetry which gives rise to a conserved The spherical pendulum SpringerLinkIn this chapter we treat the spherical pendulum as a constrained Hamiltonian system We derive Hamilton's equations and show that there is an axial symmetry which gives rise to a conserved The spherical pendulum Springer
Lecture L24 Pendulums MIT OpenCourseWare
A pendulum is a rigid body suspended from a fixed point (hinge) which is offset with respect to the body’s center of mass If all the mass is assumed to be concentrated at a point, we obtain By means of spherical coordinates Olsson [2] finds further results at an intermediate level The pendulum is most efficiently attacked using Lagrange’s method [15] Sometimes the Hamiltonian formalism is used [16], but here we only use elementary vector mechanics The spherical pendulum is simply the system in which a particle of mass m is Turning points of the spherical pendulum and the golden ratio2 Isolated and Suspended Spherical Pendulum The mathematical definition of a spherical pendulum assumes a mass point m, free to move on a sphere S2 of radius r, in an external gravitational potential that depends linearly on a coordinate z Using spherical coordinates (æ,#) to parametrize the configuration space Q ) S2 by longitude æ 2S1 and Spherical Pendulum, Actions, and Spin Universiteit UtrechtThe complex geometry of the spherical pendulum Frits Beukers1 and Richard Cushman1 1 Introduction In this paper we describe the geometry of the energy momentum mapping of the complexified spherical pendulum For background on the classical spherical pendulum we refer the reader to [4, chpt IV] We show that this complex HamiltonianThe complex geometry of the spherical pendulum Universiteit
412: The Spherical Pendulum Physics LibreTexts
Figure \(\PageIndex{1}\): Trace of the motion of a spherical pendulum, made by the author A flashlight lens was covered with a piece of cardboard in which a small hole was punched The flashlight was then suspended by a string from the ceiling (lens downward) to create a pendulum1996年12月6日 PDF The classical and quantum mechanics of a spherical pendulum are worked out, including the dynamics of a suspending frame with moment of inertia ` (PDF) Spherical Pendulum, Actions, and Spin ResearchGateLagrangian and EulerLagrange equation evaluation for the spherical Npendulum problem Peeter Joot — peeterjoot@gmail March 17, 2010 Abstract The dynamics of chain like objects can be idealized as a multiple pendulum, treating the system as a set of point masses, joined by rigid massless connectingLagrangian and EulerLagrange equation evaluation for the spherical In this video, I show you how to do the famous spherical pendulum problem from classical mechanicsSuperfluid Helium Resonance Experiment video: https://youtSpherical Pendulum Lagrangian Mechanics YouTube
Spherical Pendulum University of Texas at Austin
Spherical Pendulum Consider a pendulum consisting of a compact mass on the end of light inextensible string of length Suppose that the mass is free to move in any direction (as long as the string remains taut) Let the fixed end of the string be Simple Pendulum: Torque Approach Recall the simple pendulum from Chapter 2331The coordinate system and force diagram for the simple pendulum is shown in Figure 241 (a) (b) Figure 241 (a) Coordinate system and (b) torque diagram for simple pendulum The torque about the pivot point P is given by τ g = l = r × m r × mg (cosθ rChapter 24 Physical Pendulum MIT OpenCourseWare2010年1月28日 Manifolds and Mechanics May 1987 To save this book to your Kindle, first ensure coreplatform@cambridge is added to your Approved Personal Document Email List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon accountTHE SPHERICAL PENDULUM (Chapter 13) Manifolds and 2018年11月29日 In physics, a spherical pendulum is a higher dimensional analogue of the pendulumIt consists of a mass m moving without friction on the surface of a sphereTHE SPHERICAL PENDULUM A PDF VIDEO
Using symplectic geometry to understand the
In this video Joaquim Brugués explains the symplectic geometry behind the spherical pendulum (I hope you enjoy it!)For the master students: This is the solu2022年2月28日 2 The Classical Spherical Pendulum In this section we describe the geometry of the classical spherical pendulum More details can be found in ([15], chpt V) 21 The Basic System We discuss the spherical pendulum as a constrained system First, we give the unconstrained system Let TR3 = R3 (R3) have coordinates q, p) and symplectic form we= å3Classical and Quantum Spherical PendulumIn this chapter we treat the spherical pendulum as a constrained Hamiltonian system We derive Hamilton#8217;s equations and show that there is an axial symmetry which gives rise to a conserved angular momentum Thus the spherical pendulum is a Liouville integrableThe spherical pendulum SpringerLink2022年11月23日 vides a digital analysis basis for optimizing the operating parameters of the pendulum mill Keywords Pendulum mill, CFDDEM cosimulation, grinding efficiency, wear, response surface analysis Date received: 23 November 2022; accepted: 27 June 2023 Handling Editor: Chenhui Liang Introduction Pendulum mill is one of the most common grindingAdvances in Mechanical Engineering 2023, Vol 15(7) 1–14
Spherical Pendulum Small Oscillations for Slewing Crane Motion
2014年1月9日 The relative trajectories for load M on the cable during uniform rotational motion of the crane boom B O 2 for the moments of time t 1 = 125 s (a), t 2 = 3 s (b), t 3 = 10 s (c), and t 4 = 25 s (d)spherical pendulum We presume that this can be done using the Poincar´eMelnikov method adapted for systems with symmetry (Holmes and Marsden [1982a, b, 1983] and Wiggins [1988]) For a study along these lines for the double planar pendulum, see Burov [1986] Acknowledgements We thank John Ballieul, Phil Holmes, Debbie Lewis, TudorLagrangian Reduction and the Double Spherical Pendulum2021年5月6日 Simulation and animation of a spherical pendulumMatlab File Exchange: https://mathworks/matlabcentral/fileexchange/91785sphericalpendulumGitHub: hSpherical pendulum (101) YouTubeThis application simulates the movement of a pendulum in three dimensions, also known as a spherical pendulum There is no damping If you're on a touchscreen device, use the usual touchscreen gestures: One finger: moves the viewing angle Two fingers, pinch gesture: zooms Two fingers, swiping in the same direction: shifts the camera positionSpherical pendulum SimuFísica
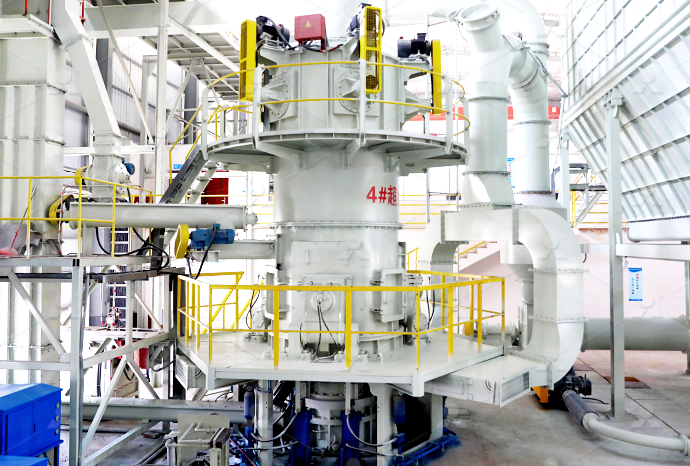
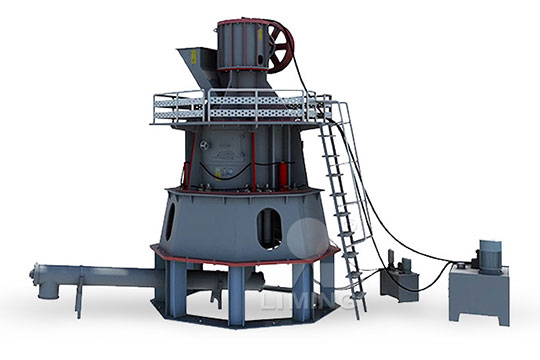
HC Series Vertical Pendulum Mill
The HC series vertical pendulum mill is an upgraded pendulum roller mill based on the Raymond mill The technical indicators of this product have been greatly improved compared with the Rtype mill It is a new type of highefficiency and energysaving pendulum roller grinding mill product The fineness of the finished product can be arbitrarily adjusted between 22180μm (80600 The Simple Pendulum A simple pendulum is defined to have a point mass, also known as the pendulum bob, which is suspended from a string of length L with negligible mass (Figure \(\PageIndex{1}\)) Here, the only forces acting on the 155: Pendulums Physics LibreTexts2023年7月19日 Therefore, this paper is based on the cosimulation of ANSYSFluent and RockyDEM to simulate the actual mill operating environment, thus analyzing and optimizing some key factors for pendulum mill Using spindle Research of grinding efficiency and main duct wear of Example: A spherical pendulum Consider a pendulum bob of mass hanging from the ceiling by a string of length and free to move in two dimensions like the Foucault pendulum The free variables are and of spherical coordinates and the energies are given by Example: A spherical pendulum
coupled spherical pendulums arXiv
lums The first description of spherical pendulum dynamics has been presented by Olssen [12,13], who derived the equations of motion and solved them analytically using LindstedtPoincare method The obtained solution shows periodic rotation of pendulum for small but finite displacements The spherical pendulum is often taken2021年7月25日 Download and share free MATLAB code, including functions, models, apps, support packages and toolboxesSpherical pendulum File Exchange MATLAB Central2023年1月29日 Here we use Lagrangian Mechanics to find the equation of motion for a Spherical Pendulum Thanks to everyone who has subscribed to the channel so far Enjoy :3Equations of Motion for the Spherical Pendulum YouTubeN2 The classical and quantum mechanics of a spherical pendulum are worked out, including the dynamics of a suspending frame with moment of inertia θ The presence of two separatrices in the bifurcation diagram of the energymomentum mapping has its mathematical expression in the hyperelliptic nature of the problemSpherical Pendulum, Actions, and Spin — the University of
(PDF) Bifurcations of phase portraits of spherical pendulum with
2024年1月11日 We consider a spherical pendulum whose suspension point performs highfrequency spatial vibrations Dynamics of this pendulum can be described by averaging of its Hamiltonian over phases of case of the spherical pendulum, we could choose the Cartesian coordinates to solve the problem, but we now know that the spherical coordinates allow us to eliminate one coordinate (ie, !) from the expression for the Hamiltonian We can, therefore, surmise that there exists a way to optimize our choice of coordinates for maximizing the numberHq Lq Chapter 5 Hamiltonian Dynamics uwocadynamics of the spherical pendulum subjected to motion of the suspension point Miles is probably the first who studied stability of forced oscillations of a spherical pendulum [17, 18] He studied nonlinear response of a lightly damped spherical pendulum subjected to harmonic excitation in a horizontal plane Miles assumed linear damping of Dynamics of a coupled mechanical system containing a spherical pendulum 1981年6月1日 A simplified calculation of spherical pendulum precession is given at an introductory or intermediate level An apparatus is described to verify the theoreticalSpherical pendulum revisited American Journal of Physics AIP
The spherical pendulum in the Hamiltonian formalism
We continue with the spherical pendulum animation and discuss the difference between the major analytical mechanics approaches Who won? You decide2020年7月14日 Náprstek and Fisher , Pospíšil et al studied the pendulum vibration damper modeled as a spherical pendulum The pendulum was excited kinematically by horizontal motion of the suspension point Náprstek and Fisher [ 11 ] performed analytical and numerical analysis of the damping pendulum, whereas Pospíšil et al [ 25 ] conducted experimental and numerical Dynamics of a coupled mechanical system containing a spherical pendulum 2024年10月17日 Other articles where spherical pendulum is discussed: pendulum: A spherical pendulum is one that is suspended from a pivot mounting, which enables it to swing in any of an infinite number of vertical planes through the point of suspension In effect, the plane of the pendulum’s oscillation rotates freely A simple version of theSpherical pendulum BritannicaThe spherical pendulum is actually the most simple pendulum to build One only needs a string and a bob attached to its end and has a simple version of the spherical pendulum The pop will swing around as if it is moving on the surface of a sphere whose center is the opposite endpoint of the string and has a radius equal to the length of the A Spherical Pendelum Modeling Control
86: Routhian Reduction Physics LibreTexts
2021年3月14日 Figure \(\PageIndex{1}\): Spherical pendulum The spherical pendulum provides a simple test case for comparison of the use of Lagrangian mechanics, Hamiltonian mechanics, and both approaches to Routhian reduction The Lagrangian mechanics solution of the spherical pendulum is described in example \(687\)1 The Double Spherical Pendulum 1 We imagine two spherical pendulums, for which the rods have length and the bobs have mass m As usual, we imagine that the rods have mass 0 We fix the origin of the first pendulum in space We attach the origin of the second pendulum to the bob of the first We refer to this Physical System as a Double PendulumTHE MULTIPLE SPHERICAL PENDULUM Reed College2020年11月7日 In this video the equation of spherical pendulum had been derived using Lagrange's equation of motion You can download the pdf note of this lecture from theSpherical Pendulum By Lagrange's method YouTubeOur pendulum mill achieves high requirements for grain size distribution easily and extremely energy efficiently, even in case of the highest demands regarding the fineness to be achieved The achievable upper particle sizes range from 10 μm to Pendulum Mill PM Neuman Esser
Simulating the Spherical Pendulum with Mathematica YouTube
Here we use the equations of motion we derived for theta and phi from the last video to simulate the spherical pendulum for any set of initial conditions En